Aha, sure enough the Rokinon 135 has a very complex backfocus for getting a flat image plane!
Earlier, I was measuring by using no glass (EAF offset = -60), and 2mm glass (EAF offset = -100 ).
Tonight, I am using the Astronomik L1 (1.1mm thick) as the glass.
Went to Alioth, Bahtinov mask focused to 12726 EAF steps.
Applied the same RA and Declination offsett as the night before, refocused, and got this:
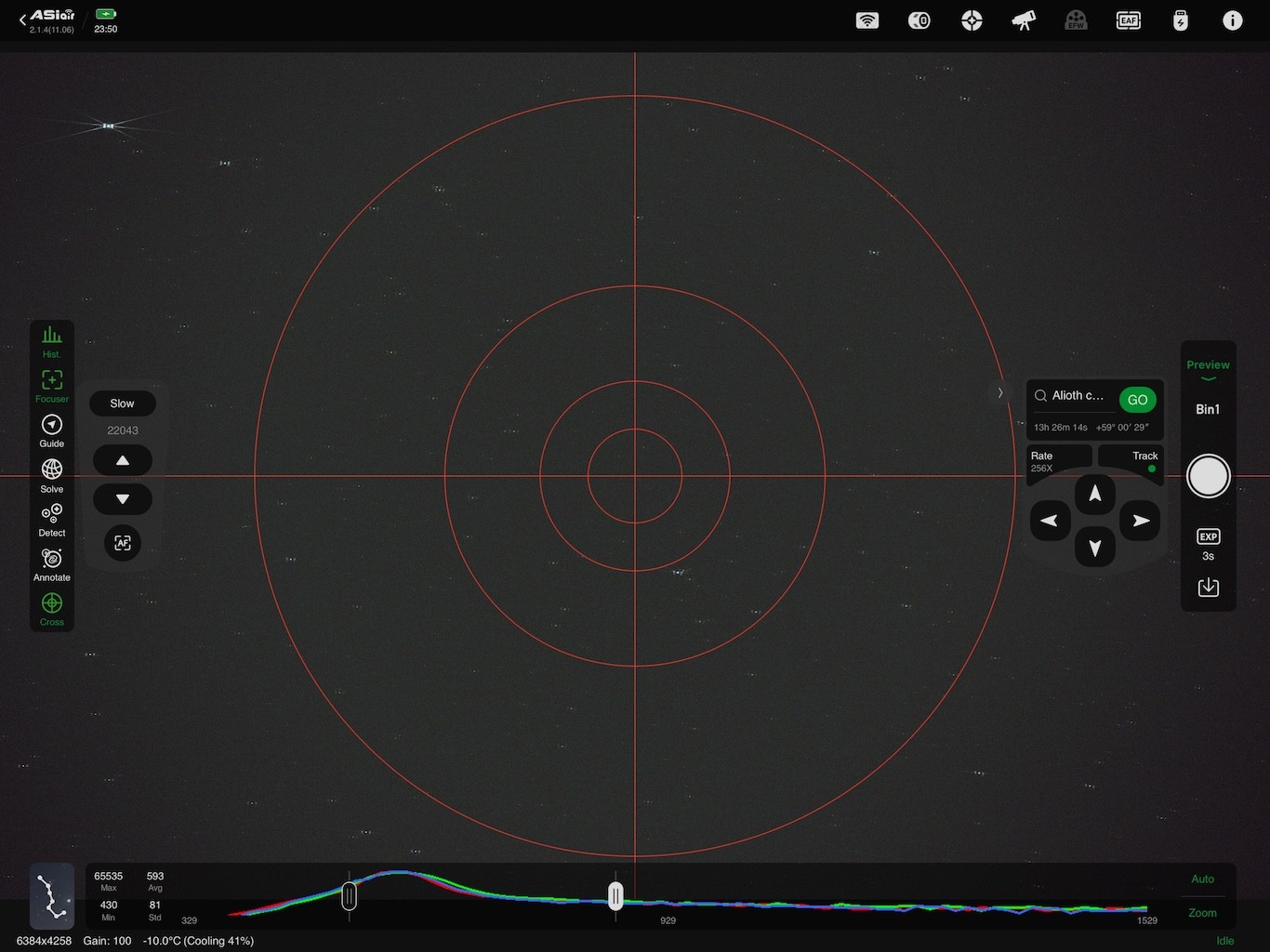
Notice that the EAF step is now 12746. The EAF had to e changed by +20 steps to refocus the corner star.
Do you notice something? The EAF offset is not in between the 0 filter and 2mm filter case. Not only that, but the EAF Offset is not only smaller (i.e., 1.1 mm glass has better flatness) but is positive.
So, if we again apply simple linear regression, this is what we see:
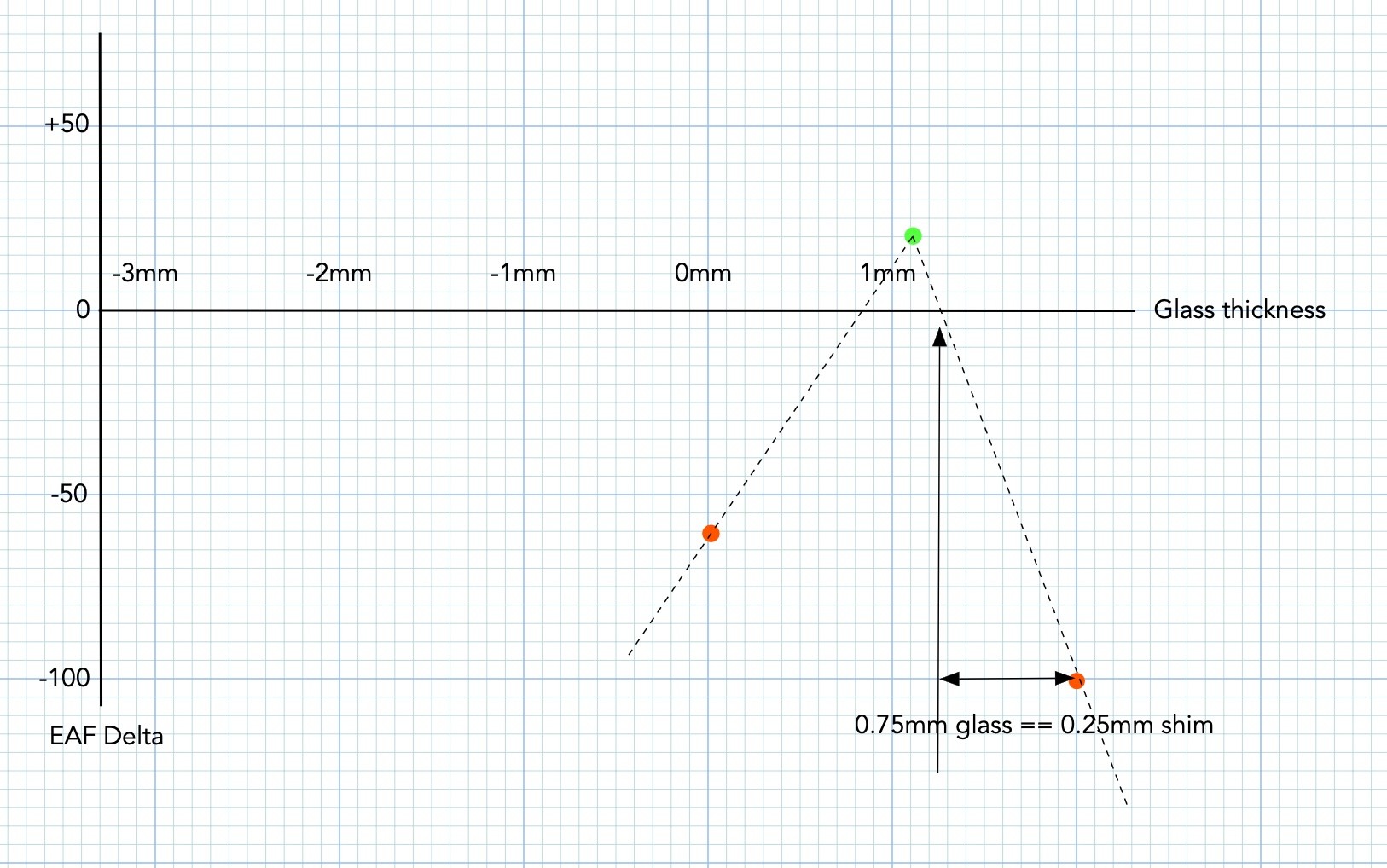
I.e., with no shimming of the backfocus, the flattest image plane happens when we use a 1.25mm thick filter (the Astronomik L1 is darn close to not needing any shimming).
And if the image plane is no more complex than we are assuming above, a 2mm glass would require only 0.25mm of shimming.
Indeed, this next (30 second) image is the magnified corner (the top left corner of the image is the top left corner of the APS-C frame, so we are looking at the farthermost corner), with no shimming, and the Astronomik L1 inserted into the ZWO EOS (sans drawer) adapter:
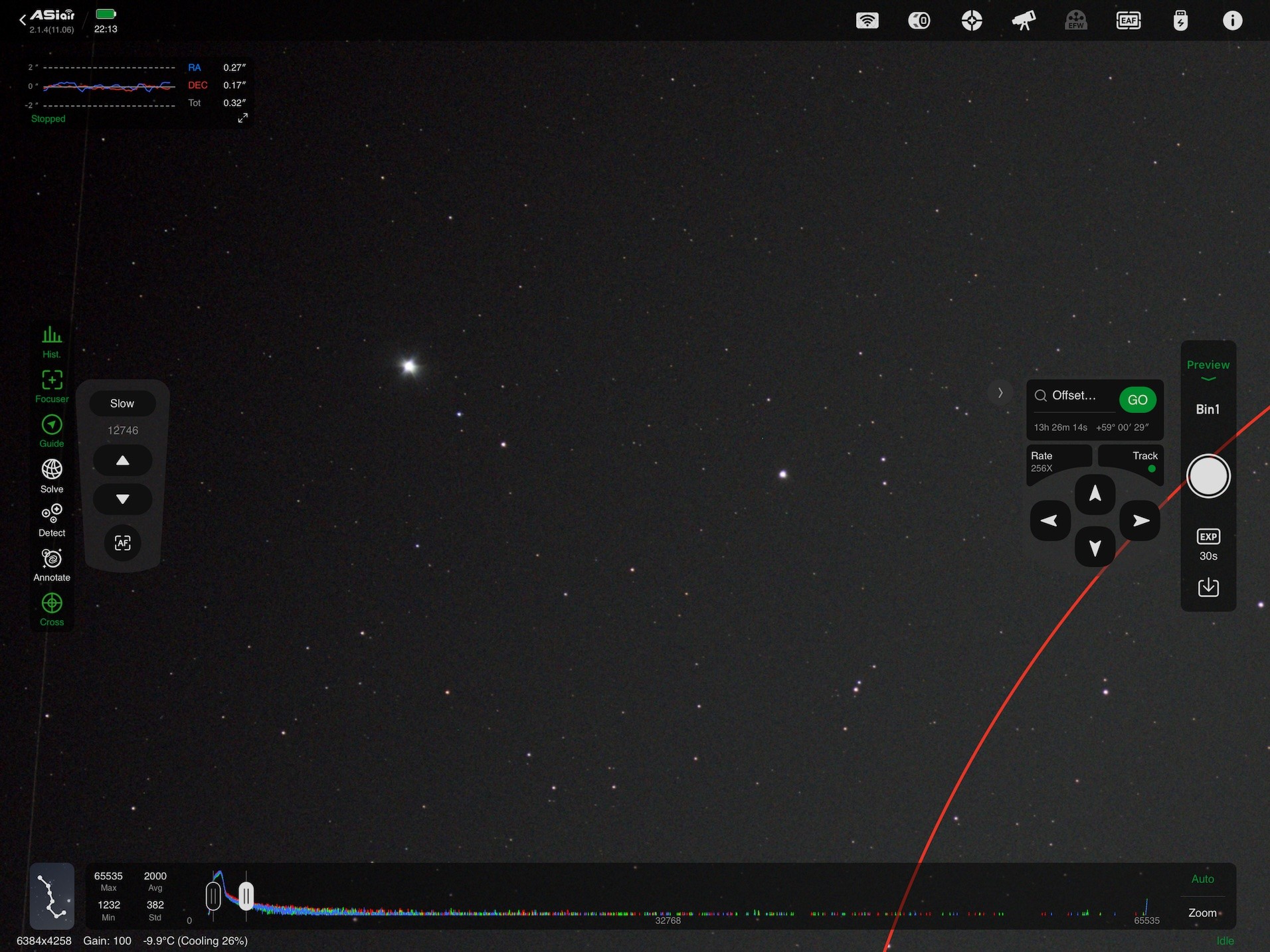
Except for the diffraction on the bright Alioth (which we know about already, I am using an aperture mask at f/2.9 using a Tiffen 52mm filter's retaining ring), notice how nice the stars are. As usual, right click on the image to see a larger image in a new window. As mentioned above, we are really close to perfect backfocus with no shimming whatsoever. Base on the "EAF Delta," and where the regression crosses 0 in the graph above, the backfocus is only off by 0.15mm worth of glass, or 0.05mm of mechanical backfocus.
Heck, I have seen worse non-round stars at the optical axis in some peoples' images!
Again, the Rikonon 135 is a darn nice lens, once we get the correct backfocus.
Chen